|
R.J. Bailey, ADAS, Gleadthorpe Land Research Centre, Mansfield, The United Kingdom, and E. Spackman, Meteorological Office, Bracknell, UK |
SUMMARY
Summer rainfall in the United Kingdom shows considerable spatial and temporal variation. The same is also true of temperature, incident solar radiation and wind speed, all of which influence evapotranspiration rates and irrigation need. Soil texture is also variable, with contrasting soils sometimes occurring in adjacent fields. A model is described which takes account of variation in soil, climate and crop growth, and produces estimates of evapotranspiration and soil moisture deficit for individual fields. This model has been incorporated into a large-scale irrigation scheduling package, using meteorological data from a network of over 200 data collection centres, together with site-specific soil and agronomic data. The package has proven successful and is currently used by over 300 farms in the United Kingdom.
Summer rainfall in the United Kingdom is highly variable. For example, records at ADAS (formerly the Agricultural Development and Advisory Service) Gleadthorpe Land Research Centre show that the average May to August rainfall occurring at the weather station is 215 mm, but this has varied between 77 (1976) and 328 mm (1969); examination of the last five years alone reveals variation of up to 50% from the long-term average (Table 1). The distribution of this rainfall has varied such that in some years it has occurred fairly evenly throughout the four-month period, but in other years one or more months were particularly dry; in 1982, for instance, May, June, July and August received 8, 64, 4 and 24% of the summer rainfall, respectively.
Irrigation scheduling based upon long-term data representing average conditions is unlikely to be satisfactory. Irrigation must be based on real-time information.
The rainfall is also often localized, varying markedly over relatively small distances. For example on 10 July 1995, 42 mm of rain was recorded at Wolverhampton, but only 2 mm at Birmingham although these two stations are only about 50 km apart.
There is also considerable spatial variation in soil type across the United Kingdom. For example, the predominant soil at Gleadthorpe Research Centre is a loamy medium sand over sand, with an Available Water Capacity (AWC) of only 9% by volume throughout the upper metre. However, within a 15 km radius there are many farms with higher AWC soils, some extending as high as 19%.
To cope with this level of variability, irrigation scheduling must not only be based on real-time information, but must be conducted on an individual field basis, and occasionally even requires parts of fields being treated separately. Growers are reluctant to undertake the numerous measurements of soil moisture that would be required, owing to the considerable amount of work involved. This has necessitated a modelling approach.
TABLE 1 - May - August rainfall during recent years at ADAS Gleadthorpe (millimetres)
|
|
May |
June |
July |
August |
Total |
|
1990 |
17 |
57 |
22 |
36 |
132 |
|
1991 |
16 |
61 |
23 |
6 |
106 |
|
1992 |
47 |
67 |
86 |
79 |
279 |
|
1993 |
57 |
84 |
83 |
42 |
266 |
|
1994 |
66 |
9 |
45 |
63 |
183 |
|
30 year average |
55 |
58 |
52 |
50 |
215 |
Evapotranspiration bulletins, based upon Penman calculations, have been issued in the United Kingdom at various times by both the Ministry of Agriculture, Fisheries and Food (MAFF) and the national Meteorological Office. Growers were issued instructions on keeping a water balance for individual fields (MAFF, 1954), using the regularly published evapotranspiration estimates for their region, together with their own rainfall and irrigation amounts. Improvements in subsequent years led to the development of the Meteorological Office Rainfall and Evapotranspiration Calculation System MORECS, (Thompson et al., 1981). This produces weekly averages for each of 190 grid squares, of 40 × 40 km dimension, superimposed over the whole of Great Britain, using daily data from a network of more than 200 synoptic weather stations. Simultaneously, the Ministry of Agriculture recommended improvements in the water balance calculation (for a full description see Bailey, 1990).
Regardless of the above developments, the system was not popular with growers. Although the water balance calculations required were simple, the need for a separate estimation of soil moisture deficit (SMD) for each field resulted in a lengthy task for those growers with numerous fields to manage. In addition, whilst the technique was fairly reliable with crops which had full leaf cover and were well supplied with water, the simple calculations inevitably produced inaccuracies when used on crops with a small leaf area or when used during times of insufficient irrigation leading to a drying soil and water stress.
It became obvious that, for irrigation scheduling to be adopted widely by farmers, it was necessary to develop an accurate system that was more convenient for them to use. As a result, ADAS and the Meteorological Office have jointly developed an irrigation scheduling model that takes account of local variation, but is almost entirely computer driven and provides regular irrigation advice.
OPERATIONAL DETAILS
Meteorological data
The model requires sufficiently detailed meteorological data to execute Penman calculations. Such measurements are impractical on commercial farms, and the data is collected from a network of over 200 synoptic stations administered by the Meteorological Office, and most dense in the areas of intensive irrigation. These data are transferred each day, via a permanent on-line data link, to a centralized mainframe computer on which the model is stored. Using a precisely identified location for each farm, the model interpolates between nearby stations (up to eight may be used) to establish site-specific data for each climatic variable. The interpolation procedure produces a weighted mean, using a lower weighting with increasing distance from the farm. This procedure not only prevents undue bias by an occasional incorrect value but also copes with missing data.
Start-of-season farm data
At the start of the season, the soil of each field is examined by a skilled adviser and the AWC estimated. These details are also stored for use in subsequent seasons. Information on the crops to be grown in each field is provided by the grower at this time.
Regular farm data
The large variation in rainfall is such that although interpolation from the synoptic network data is possible, it is far less reliable than on-site data. Growers are therefore encouraged to take daily readings from their own rain gauge, and communicate these at regular intervals to their local ADAS adviser by telephone, telefax, etc. At the same time, details are supplied of all irrigations applied since the last communication together with relevant agronomic data such as crop planting dates, emergence dates, and leaf cover percentage if available. Upon receipt of this data, the model is run and updated advice on irrigation scheduling for each individual field is forwarded to the grower.
Updating this information and running the model can be done as often as required but weekly is the norm.
MODEL DESCRIPTION
A full description of the calculations used in the model will be reported elsewhere, but it is appropriate to describe here the general outline and, in particular, the manner in which variations in weather, soil and plant growth are accounted for. The following steps are involved: (i) calculation of potential evapotranspiration for a reference crop (PEr) on each farm; (ii) use of the PEr estimates to drive growth sub-models for crops in each individual field on the farm; (iii) calculation of potential evapotranspiration for each field (PEc) taking crop growth into account; (iv) estimation of actual evapotranspiration (AEc) and soil moisture deficit (SMD) within each field; and (v) production of irrigation schedules.
The reference crop is considered to have the following characteristics: leaf area index 5; canopy resistance 40 sm-1; height 0.3 m; albedo 0.25. The calculation of PEr uses a modified version of the Penman-Monteith equation as found in MORECS,
The main components of the equation are:
D slope of saturation vapour pressure curve
RN net radiation
G heat energy absorbed and stored in the soil
r cp (SVP-VP)/ra an aerodynamic term including wind speed and vapour pressure deficits
(1 + rs/ra) a term incorporating crop and aerodynamic resistance
For further explanation of this equation see Thompson et al (1981).
Crop growth sub-models are then run for each field to estimate percentage leaf cover, leaf area index (LAI), crop height, rooting depth, aerodynamic canopy resistance and surface resistance of the crop. These sub-models are based on empirical relationships between each parameter and PEr estimates, having been calibrated for all the major crop species grown in the United Kingdom. The linking of crop growth to PEr allows for the effects of local variation in temperature, solar radiation, etc., on crop growth to be taken into account. Any details provided by the grower regarding leaf cover development can be used to check the sub-models and adjust them, or even override them, if required. This may be relevant in instances of unexpected defoliation as a result of pest, disease or herbicide damage, for instance.
The model then recalculates the Penman equation using updated values for each crop growth parameter, such as aerodynamic and surface resistances, to produce PEc estimates for each individual field.
FIGURE 1 - Actual/potential evapotranspiration ratio plotted as a function of soil moisture tension for five values of potential evapotranspiration

The actual evapotranspiration (AEc) for each field is estimated from the PEc as follows:
AE
c = k PEc
where k is a coefficient dependent on the soil moisture deficit and the potential transpiration rate, equal to or approaching unity when the soil is near field capacity, and decreasing as the soil dries. Makkink and van Heemst (1956) and Denmead and Shaw (1962) showed how k decreases as PEc rates increase, a further source of variation in the changeable climate in the United Kingdom. In this model, an empirical relationship has been calibrated to fit the data of Denmead and Shaw (1962) closely and is used to estimate k. When PEc is greater than 1.4 mm day-1,
k
= [(PEc - 1.4)/6.3]4.25 y -1.24
where y approximates to soil moisture tension (in bars1). When PEc is 1.4 mm day-1 or less, k = 1. Figure 1 shows values of k produced by the model, for a selection of PEc values, as soil moisture tension increases.
1
1 bar = 102kPa
The estimates of AEc are used to derive SMD values for each field by means of a simple water balance. During times of excess rainfall or irrigation, the water balance includes functions to estimate drainage amounts (based on amount of excess water and soil characteristics) and runoff (based on amount of excess water, soil characteristics and slope of field).
The model receives daily updated weather forecasts from the meteorological office, which are applied to the estimates of SMD and used to derive forecast values for up to two weeks ahead. Bailey (1990) has published a list of critical SMD values for the United Kingdom's major crops on a complete range of soil types, the critical SMD being that at which yield or quality are affected. These are used with the SMD forecasts to estimate the next date by which irrigation is required.
FIGURE 2 - Comparison between measured (·) and modelled (___) soil water change for potatoes. Vertical bars represent the standard error for measured values
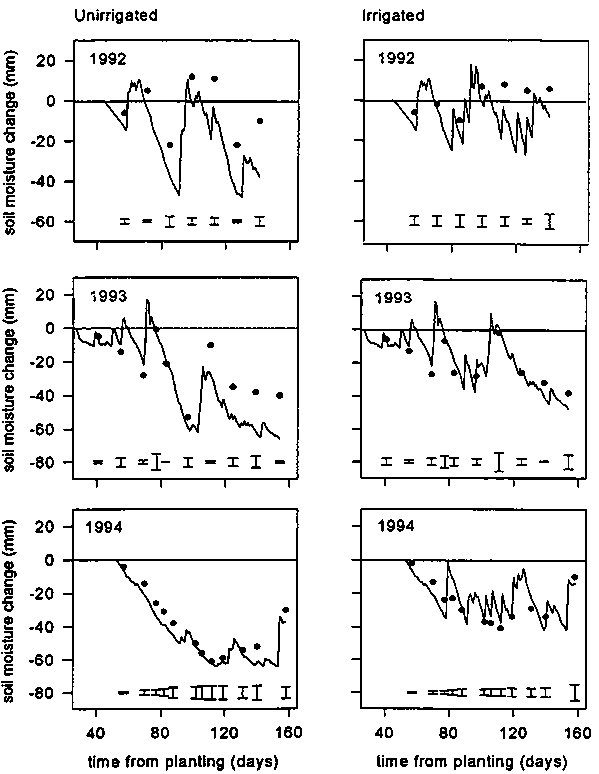
FIGURE 3 - Comparison between measured (·) and modelled (___) soil water change for sugar beet. Vertical bars represent the standard error for measured values
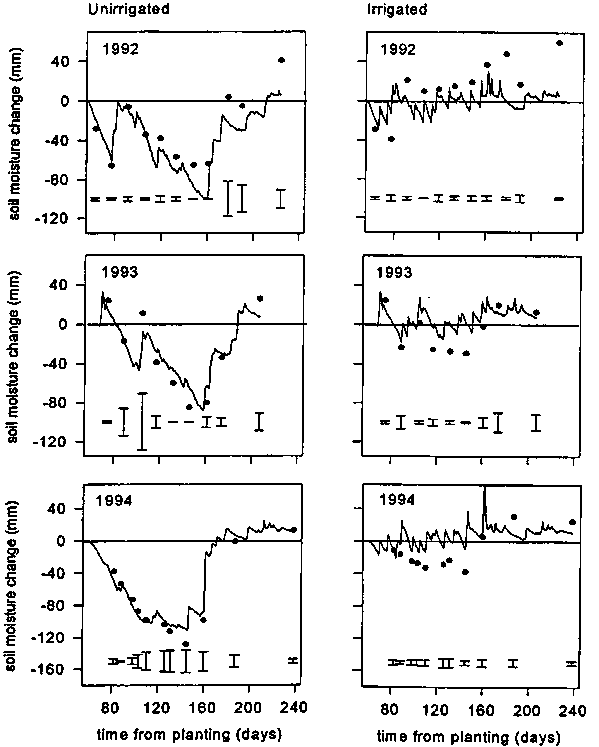
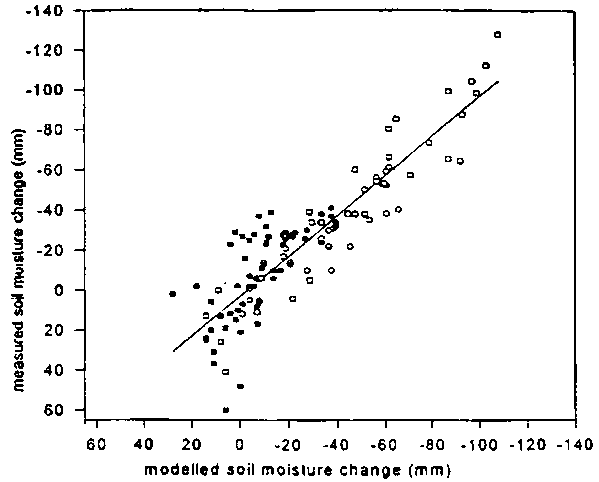
FIGURE 4 - Relation between measured soil water change and model estimates for potatoes (O) and sugar beet (_). Open symbols represent unirrigated or drought treatments, closed symbols represent irrigated treatments. The fitted line accounts for 80% of the variation, and is of the form of y = 3.00 + 0.99 x.
VERIFICATION AND FIELD TESTING OF MODEL
The model was used to estimate soil moisture changes under irrigated and non-irrigated potatoes and sugar beet at Gleadthorpe Land Research Centre over several years, and tested against neutron probe measurements. Data from 1992-1994 are presented in Figures 2 and 3. These comparisons can be expressed qualitatively by calculating a bias (i.e., the mean difference between the measured and estimated change) and a root mean square (RMS) of the differences between them (Johns and Smith, 1975). The mean bias value recorded here was only -3 mm and the mean RMS only 16 mm. Gardener and Field (1983) suggested that an RMS of 20 mm was a reasonable dividing line between a good fit or otherwise in modelling soil moisture changes. The value of 16 mm obtained here suggests that the model performed reasonably well. This is supported by the linear regression of measured versus modelled data (Figure 4), which indicated a highly significant relation (r2 = 0.80), a slope of 0.99 and an intercept of only 3 mm.
The model has also been used to schedule irrigation on a large number of commercial farms for several years, and has proved successful. Currently, over 300 farms in England and Wales are using it to manage their irrigation for a wide range of crops and soil types.
ACKNOWLEDGEMENTS
The authors thank the Ministry of Agriculture, Fisheries and Food of the United Kingdom for funding the work.
REFERENCES
Bailey, R.J. 1990. Irrigated Crops and Their Management. Farming Press, Ipswich.
Denmead, O.T. and Shaw, R.H. 1962. Availability of soil water to plants as affected by soil moisture content and meteorological conditions. Agronomy Journal 54, 384-390.
Gardener, C.M.K. and Field, M. 1983. An evaluation of the success of MORECS, a meteorological model, in estimating soil moisture deficits. Agricultural Meteorology 29: 269-284.
Johns, G.G. and Smith, R.C.G. 1975. Accuracy of soil water budgets based on a range of relationships for the influence of soil water availability on actual water use. Australian Journal of Agricultural Research 26: 871-883.
MAFF. 1954. The calculation of irrigation need. MAFF Technical Bulletin No. 4, HMSO, London.
Makkink, G.F. and van Heemst, H.D.J. 1956. The actual evapotranspiration as a function of the potential evapotranspiration and the soil moisture tension. Netherlands Journal of Agricultural Science, 4: 67-72.
Thompson, N., Barrie, I.A. and Ayles, M. 1981. The Meteorological Office rainfall and evaporation calculation system: MORECS (July 1981), Meteorological Office Hydrological Memorandum No 45.