|
I. Varlev and P. Dimitrov, Scientific Institute for Irrigation and Drainage, and Z. Popova, Institute for Soil Science and Agroecology, Sofia, Bulgaria |
SUMMARYThe objective of this paper is to present practical rules for optimal irrigation management under limited water supply at farm level. A basic objective function (Eq. 12) relating yield to water deficit to full crop satisfaction is derived from 'yield-evapotranspiration' and 'yield-irrigation depth' relationships. For different soil and climate conditions, maximum yield was obtained with a limited quantity of irrigation water. The research is extended to practice by formulating the following rules: the farmer should satisfy 75-80% of the required irrigation depth starting from the most sensible to the least sensible phases of development. It is advisable to keep relative evapotranspiration over 0.7 during the initial two stages so that crop development is not stressed during the next period. Usually the yield obtained under rainfed conditions in regions with a semi-humid climate (such as Bulgaria) is 40-60% of the regional productivity potential. If two-thirds of the required water is available, one could obtain about 90-95% of the maximum yield; when applying only 50% of the water required, the yield could be 80-85% under optimal irrigation management.
Crop water requirements cannot be fully met in many regions of the world when water availability is insufficient. Different crops respond in a specific manner to the deficit of water depending on the crop development stage. A contemporary review of the different existing equations is presented by Pereira (1994). Jensen (1968) supposes a multiplicative equation for reading crop sensitivity during individual crop growth periods. Cumulative equations have been proposed by Hiler and Clark (1971) and Stewart et al. (1977). These models have also been latter proposed by FAO (Doorenbos and Kassam, 1979).
Many papers seeking to achieve optimal use of insufficient irrigation water have been published during the last two decades, namely Mechandjieva et al. (1978), Hanks (1983), Howell et al. (1971). A solution for optimal water deficit related to semi-arid climate is presented by Ghahraman and Sepaskhan (1994).
The objective of the present study is to establish practical rules for optimal irrigation management under limited water supply at farm level, where precipitation contribute significantly to crop water use.
BACKGROUND ON YIELD-WATER RELATIONSHIPS
Crop response to the deficit of water over the different crop development stages is accounted for by the following multiplicative equation derived by Jensen (1968).
, (1)
with
yi* = Yi/Ymax, (2)
ej,i* = ETj,i/ETmax,i, (3)
where:
yi* relative yield for treatment j,
Yj actual yield for treatment j (kg ha-1),
Ymax maximum yield (kg ha-1),
ej,i* relative evapotranspiration at growth period i for treatment j,
ETj,i actual evapotranspiration at growth period i for treatment j (mm),
ETmax,i maximum evapotranspiration at growth period i (mm).
Doorenbos and Kassam (1979) adopted the model of Stewart et al. (1977). The Stewart phasic model is:
, (4)
l i, and b i, in Eq. (1) and (4) are the crop sensitivity factors for period i.
Field trials to evaluate the response of maize to water deficit during a particular growth period were carried out with maize hybrid H708 during a period of seven years from 1984 to 1990 (Varlev et al., 1992). The irrigation season is divided into three growth periods. The first period is from the ' 10th leaf to tasseling'; over the years it occurred on average between 25 June and 15 July. The second period is from 'tasseling to milky ripening' (16 July to 31 July) and the third is from 'milky ripening to waxy ripening' (1 August to 31 August).
The results of 83 field trials have been analysed. Experimentally-based relative evapotranspiration ranged from 0.2 to 1.0 (compared with 0.5 - 1.0 in Doorenbos and Kassam, 1979).
Four equations have been tested. For the Jensen relationship (Eq. 1), the coefficient of determination is R2 = 0.716. The highest coefficient of determination, R2 = 0.908, is obtained with the linear equation (Varlev et al., 1992)
, (5)
where:
Ki coefficient of crop response to water deficit during a particular i. period,
D y*i relative yield loss (1 - yi*) caused by water deficit during stage i.
As a result of seven years of experiments (Varlev et al., 1992), the values of Ki over the three maize growth periods considered are respectively: K1=0.29, K2= 0.77 and K3=0.18.
Equations (4) and (5) have similar structure. However, using ei,j*= ETj,i/ETmax,i in Eq. (5) makes the denominator ETmax,i, which takes into consideration the different length of the periods i, while in Eq. (4) only ETmax is considered.
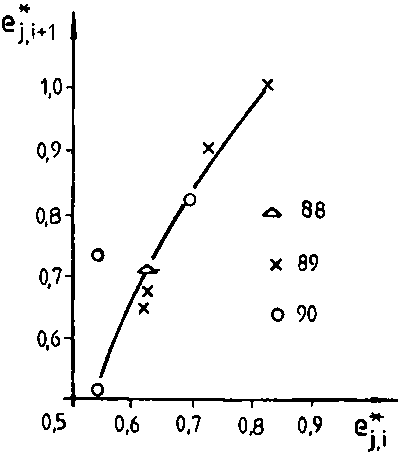
Some investigations prove that water deficit during a particular period i influences the maximum crop water use during the next period, i+1, even if available water is non limiting. In Figure 1, relative evapotranspiration during period i+1 is plotted against relative evapotranspiration during the period i. This shows that when relative evapotranspiration during period i is less then 0.7 it induces stress on the crop during next period i+1. Information from Figure 1 should be taken into consideration when scheduling irrigation with water deficits.
FIGURE 1 - Influence of stress induced during the growth period i on the relative evapotranspiration during period i+1 for maize
OPTIMIZATION
Relative evapotranspiration for period i and treatment j, e*j,i (Eq. 5) can be considered as a sum:
e*j,i = e*sw,i + e*pre,i + e*irr,j,i= e*rain,i + e*irr,j,i, (6)
where:
e*sw,i relative evapotranspiration supplied by the available soil water at beginning of period i,e*pre,i relative evapotranspiration supplied by the precipitation during period i,
e*irr,j,i is relative evapotranspiration due to the applied irrigation water during the period i for the treatment j.
e*rain,i = e*sw,i + e*pre,i
corresponds to the contribution of natural resources to crop water use over the different crop growth periods i.
Relative evapotranspiration due to irrigation could be expressed by:
e*irr,j,i = Wj,i/ETmax,i . ti, (7)
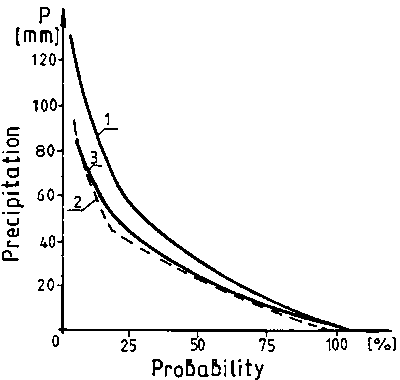
where Wj,i is the applied net depth of water for treatment j, over the i period with ti duration. In regions' with desert and arid climate the influence of precipitation and soil water on evapotranspiration is negligible and esw,i = epre,i » 0. In regions with semi-humid climate the contribution of natural water supply erain,i is significant. Probability curves resulting from long term observations of precipitation over the three growth periods considered for south Bulgaria are presented in Figure 2.
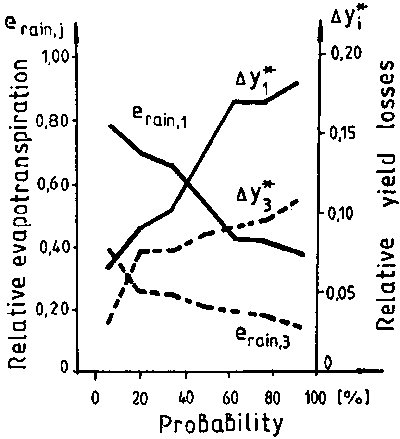
Figure 3 is based on seven-year experiments with grain maize under local climate and soil conditions without irrigation. The component esw,i is derived from the soil water content at the beginning of each period i. The values of e*rain,3 could be higher than those in Figure 3 when irrigation is applied during the first and second periods.
To establish optimal distribution of insufficient irrigation water Wav = a Wmax, the relative evapotranspiration, e*j,i has to be expressed by the relative irrigation depth, w*j,i.
w*j,i = Wj,i/Wmax,i, (8)
where:
Wj,i net depth of water applied with treatment j during period i (mm),
Wmax,i net depth of water during period i which leads to maximal yield (mm).
FIGURE 2 - Rainfall probability curves for Calapica, south Bulgaria for three maize growth stages: 1: 10th leaf to tasselling; 2: tasselling to milky ripening; 3: milky to waxy ripening.
When the reduction of irrigation depths is uniform over the whole irrigation season, the following equation can be used (Varlev, 1994):
y*i = y*rain + 2(1 - y*rain)w*j - (1 - y*rain)w*j2, (9)
where
y*rain relative yield under rainfed conditions (=Yrain/Ymax),
w*j relative irrigation depth for treatment j (= Wj/Wmax),
Wj irrigation depth for treatment j (mm),
Wmax irrigation depth which leads to maximal yield (mm).
FIGURE 3 - Probability curves for relative evapotranspiration secured from rain and relative yield losses during two growth periods for maize.
Results from field trials are shown in Figure 4 when water deficit is significant only over the first stage, thus when (e*2 = e*3 = 1.0). Under these conditions Eq. (9) becomes:
y*i,1 = y*rain,1 + 2(1 - y*rain,1)w*j,1 - (1 - y*rain,1)w*j,12, (9a)
and Eq. (5) is simplified into:
y*i,1 = 1 - K1 + (1 - e*i,1), (5a)
Relative yield under rainfed conditions when e2 = e2= 1.0 is then:
y*rain,1 = 1 - K1 + K1e*rain,1, (10)
If Eq. (5a) is set equal to Eq. (9a), having in mind Eq. (10), the result is:
e*i,1 = e*rain,1 + 2(1 - e*rain,1)w*j,1 - (1 - e*rain,1)w*j,12, (11)
Eq. (11) also yields relative evapotranspiration e*j,i when relative irrigation depth w*j,i is known.
By substituting Eq. (11) in Eq. (5), the following equation is obtained:
, (12)
The following balance equation over the whole irrigation season holds:
W1 + W2 + W3 = Wav = a Wmax, (13)
where a (0,1) is a ratio of available water Wav (mm) to optimal irrigation depth for maximum yield, Wmax (mm), and Wi are the irrigation depths (mm) applied during the three growth periods.
Optimum distribution of available irrigation water Wav = a Wmax will be achieved when y*j,i (Eq. 12) reaches a maximum.
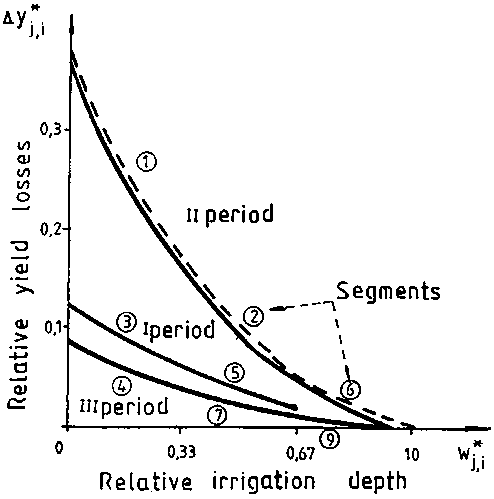
Equation (12) calculates yield in dependence of applied water, w*j,i, and requires a non-linear optimization approach. A linearization, D y*i, of the objective function can be adopted using successive linear segments as presented in Figure 5. The number of segments is accepted to be three for each period (i = 1, 2, 3). The slope of each segment corresponds to the specific yield losses D y*i, per unit of applied water deficit, which supports selection of strategies for obtaining maximum yields.
FIGURE 4 - Relative yield versus relative evapotranspiration and relative water application for maize during the first growth period - 1990
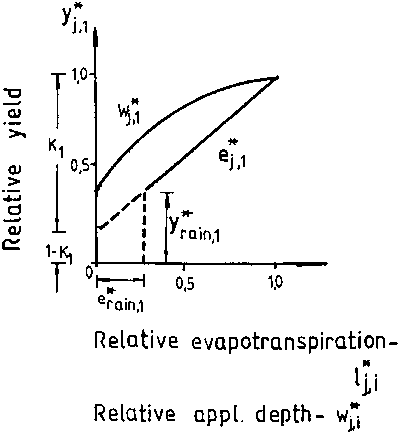
FIGURE 5 - Relative yield losses versus relative irrigation depth for the three growth periods of maize: linearization of Eq. (12); e*rain,1 = 0.55; e*rain,2 = e*rain,3 = 0.50
APPLICATION IN IRRIGATION SCHEDULING PRACTICE
Tables 1, 2 and 3 illustrate the possible strategies when optimizing irrigation scheduling under deficit irrigation.
Table 1 contains the input data for this example. Table 2 shows the procedure followed to achieve the maximum yield when the available net applied water Wav is 70% of the required water Wmax for the whole season. Strategy 1 (Table 2) corresponds to full satisfaction of water requirements (w*j,i = 1.0) during the most sensitive phases (the first, k1=0.29 and the second, k2=0.77). The remaining W3=5.8 mm are applied during the third period (k3=0.18). This strategy (w*1=w*2= 1 and w*3=0.08) enables 0.924 of the maximum yield to be obtained.
Strategy 2 is related to 90% satisfaction of the crop water requirements during the most sensitive phases (first and second). The remaining 20.6 mm are applied during the third period (w*1=w*2=0.9, w*3=0.29 and y* = 0.949). For the last strategy (4 in Table 2) the available irrigation water is equally applied over the season (w*i = 0.7w*max,i). Strategy 3 results in the highest yield when 80% of required water is applied for the two most sensitive periods.
TABLE 1 - Input data to the example given in Table 2 for a maize crop
|
Characteristics |
Growth periods |
Total |
|||
|
1 |
2 |
3 |
|||
|
Period duration |
(day) |
20 |
16 |
31 |
67 |
|
ETmax |
(mm/day) (mm) |
5.50 |
7.00 |
3.30 |
324 |
|
ETrain |
e*rain,i |
0.55 |
0.50 |
0.50 |
167.5 |
|
ETirr,max |
e*irr,i |
0.45 |
0.50 |
0.50 |
156.5 |
|
Coefficients of sensitivity |
Ki |
0.29 |
0.77 |
0.18 |
1.24 |
Note: Maximum irrigation depths Wmax,i are set equal to 1.4 ETirr,i.
TABLE 2 - Strategies for scheduling maize crop irrigation for Wav = 0.7 Wmax (see Table 1 for input data)
|
Strategy ymax,i |
Parameters |
Periods |
Relative yield losses |
Relative yield |
|||
|
1 |
2 |
3 |
|||||
|
Rainfed |
D y*i |
0.131 |
0.385 |
0.090 |
0.606 |
0.394 |
|
|
1 |
1.00 |
Wj,i (mm) |
69.3 |
78.4 |
5.8 |
|
|
|
w*j,i |
1.00 |
1.00 |
0.08 |
|
|
||
|
D yj,i |
0.00 |
0.00 |
0.076 |
0.076 |
0.924 |
||
|
2 |
0.90 |
Wj,i (mm) |
62.4 |
70.6 |
20.6 |
|
|
|
w*j,i |
0.90 |
0.90 |
0.29 |
|
|
||
|
D yj,i |
0.0013 |
0.0039 |
0.0457 |
0.051 |
0.949 |
||
|
3 |
0.80 |
Wj,i (mm) |
55.4 |
62.7 |
35.4 |
|
|
|
w*j,i |
0.80 |
0.80 |
0.49 |
|
|
||
|
D yj,i |
0.006 |
0.015 |
0.023 |
0.044 |
0.956 |
||
|
4 |
0.70 |
Wj,i (mm) |
48.5 |
54.9 |
50.1 |
|
|
|
w*j,i |
0.70 |
0.70 |
0.70 |
|
|
||
|
D yj,i |
0.012 |
0.035 |
0.008 |
0.055 |
0.945 |
||
Remarks: Ymax,i [0,1] level of satisfaction of irrigation water requirements during the most sensitive periods i.
Table 3 gives the relative yield (Eq. 12) when available water varies within the range a = 0.2 -0.8. The strategies of maximum level of satisfaction of irrigation water requirements during the particular periods (g max,i) range from 1.0 to 0.2 (Table 3, column 1). The maximum relative yields are given in bold for each a.
An optimization similar to that in Table 3 was performed for different soil and climatic conditions. (e*1 = 0.3...0.8; e*2 = e*3 = 0.0...0.7). Resulting maximum yields are given by the curves in Figure 6. All results from optimization confirm the general rule that maximum yields under limited water supply correspond to strategies that satisfy 75-80% of the irrigation water requirements, when relative evapotranspiration during the first and second growth periods should be kept over 0.7.
Following this practical rule, actual yields differ by less than 1% from the mathematically computed maxima (Tables 2 and 3).
TABLE 3 - Relative yields from different water availability [a = 0.2, 0.8] and several strategies for satisfying irrigation water requirements of maize when e*rain,1 = 0.55; e*rain,2 = e*rain,3 = 0.50; y*rain = 0.394
|
Ymax,i |
Availability of irrigation water a = Mav/Mmax | ||||||
|
|
0.20 |
0.30 |
0.40 |
0.50 |
0.60 |
0.70 |
0.80 |
|
1.00 |
0.705 |
0.770 |
0.812 |
0.871 |
0.903 |
0.924 |
0.966 |
|
0.95 |
0.705 |
0.770 |
0.824 |
0.877 |
0.905 |
0.939 |
0.975 |
|
0.90 |
0.705 |
0.770 |
0.832 |
0.881 |
0.904 |
0.949 |
0.980 |
|
0.85 |
0.705 |
0.770 |
0.838 |
0.883 |
0.913 |
0.955 |
0.981 |
|
0.80 |
0.705 |
0.775 |
0.841 |
0.881 |
0.920 |
0.956 |
0.976 |
|
0.75 |
0.705 |
0.780 |
0.842 |
0.877 |
0.923 |
0.953 |
|
|
0.70 |
0.705 |
0.783 |
0.839 |
0.879 |
0.921 |
0.946 |
|
|
0.65 |
0.705 |
0.782 |
0.834 |
0.878 |
0.914 |
|
|
|
0.60 |
0.705 |
0.779 |
0.826 |
0.873 |
0.903 |
|
|
|
0.55 |
0.704 |
0.773 |
0.821 |
0.863 |
|
|
|
|
0.50 |
0.700 |
0.764 |
0.813 |
0.849 |
|
|
|
|
0.45 |
0.693 |
0.753 |
0.800 |
|
|
|
|
|
0.40 |
0.684 |
0.741 |
0.782 |
|
|
|
|
|
0.35 |
0.671 |
0.724 |
|
|
|
|
|
|
0.30 |
0.656 |
0.703 |
|
|
|
|
|
|
0.25 |
0.637 |
|
|
|
|
|
|
|
0.20 |
0.613 |
|
|
|
|
|
|
Ymax,i: see note at bottom of Table 2.
FIGURE 6 - Relative yield of maize as a function of the relative available irrigation water (a = Wav/Wmax) for different climates: 1) e*rain,1 = 0.80; e*rain,2 = 0.79; e*rain,3 = 0.50; 2) e*rain,1 = 0.55; e*rain,2 = e*rain,3 = 0.50; 3) e*rain,1 = 0.50; e*rain,2 =: e*rain,3 = 0.20; 4) and 4') e*rain,1 = 0.30; e*rain,2 = e*rain,3 = 0.0 (4' with uniform distribution of irrigations during the season)
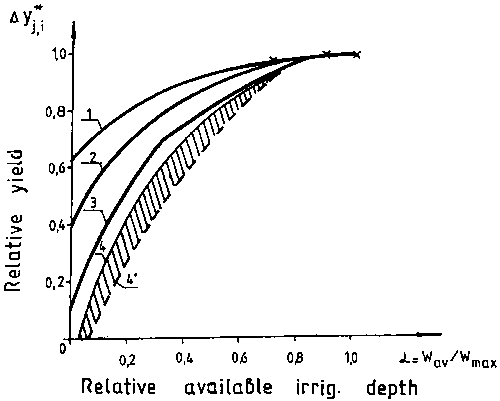
The curve 4' in Figure 6 concerns arid condition when the irrigation water is uniformly distributed over the season. The shadow zone shows the increase in yield (10-30%) which could result from optimization. These results could be extended to practice, namely when high water costs would lead to an economic optimal application depth, Wopt, lower than Wmax.
Figure 6 illustrates other cases. When the available water resources satisfy about two-thirds of the irrigation water requirements (a » 0.7), and since the late season period is the least sensitive to water deficit (K3=0.18 for maize), the best strategy is to satisfy irrigation water requirements during the first and second growth periods, adopting w*j,i = 0.8. Thus, only the remaining water would be applied during the third period.
Adopting this strategy, yield losses are minimized, while soil water reserves are depleted at the end of the season, which helps to limit nutrients leaching in autumn and winter.
When available water covers about 50% of the irrigation water requirements, these should be satisfied in 75-80% during the most sensitive period (the second), and partially during the next sensitive one (the first). The graphs in Figure 6 show that 85-87% of the maximum yield could then be obtained (curve 3, Figure 6).
If the available water satisfies only one-third of the irrigation water requirements, all the water should be applied during the most sensitive period (the second). However, in very dry years, a part of these limited water resources should be applied during the first period so that the relative evapotranspiration is kept above 0.7. It is then possible to achieve 70-78% of the maximum yield (curve 2, Figure 6).
CONCLUSIONS
The research on scheduling irrigation with limited water supply provides for the following conclusions:
1. In the regions with a semi-humid climate (such as Bulgaria) the average yield obtained under rainfed conditions is 40 to 60% of the potential yield, Ymax2. For such conditions, the following practical rules for maximizing yields under water deficit can be formulated:
· the irrigation water requirements should be satisfied in about 75-80% starting from the most sensitive to the less sensitive crop growth periods;· it is advisable to keep relative evapotranspiration above 0.7 during the first and second growth periods so that crop development is not stressed during the next period.
3. If two-thirds of the required water is available, one could obtain about 90-95% of the maximum yield, while if the water is half that required, the yield could be 86-87% under optimum irrigation management. In the first case this could be achieved by giving priority to irrigation during the first two crop stages, while in the second case this corresponds to applying limited water in the first period and satisfying crop demand during the second one.
REFERENCES
Doorenbos, J. and Kassam, A. 1979. Yield response to water. FAO Irrigation and Drainage Paper 33. Rome.
Ghahraman, D. and Sepaskhan, A.K. 1994. Optimum Water Deficit in Irrigation Management in Semi-Arid Region in Iran. 17th Europ. Reg. Conf. on ICID, Varna, May, pp. 127-134.
Hanks R.J. 1983. Yield and water use relationships: an overview. In: Limitation in Efficient Water Crop Production. P.I. Tailor (ed.). Amer. Soc. of Agron., Madison.
Hiller, E.A. and Clark, R.N. 1971. Stress day index to characterize effects of water stress on crop yields. Transactions of the ASAE, pp. 757-761.
Howell, T. and Hiller, E.A. 1971. Optimization of water use efficiency under high frequency irrigation. Transactions of the ASAE, pp. 873-878.
Jensen M.E. 1968. Water Consumption by Agricultural Plants, Water Deficits and Plant Growth. T.T. Kozlowsky (ed.). Academic Press, New York. Vol. II, pp. 1-22.
Mehandjieva, A., Lazarov, R. and Ugartchinsky, S. 1978. Grading the crops for irrigation scheduling of insufficient available water. Water Management, No. 2, Sofia. pp. 22-31 (in Bulgarian).
Pereira L.S. 1994. Yield Response to Water as Affected by Irrigation Scheduling. General report of the 17th Europ. Reg. Conf. on ICID, Varna, Bulgaria, May. pp. 1-19.
Stewart J.T. et al. 1977. Optimizing Crop Production through Control of Water and Salinity Levels in the Soil. Utah Water Lab. PRW 6-161-1.
Varlev, N. Kolev, N. and Kirkova, J. 1992. Modelling the Impact of Water Deficit During Particular Phases of Crop Development on Yield Production. Technical Sciences, Vol.1, Sofia, pp. 50-57 (in Bulgarian).
Varlev, I., Kolev, N. and Kirkova, J. 1994. 'Yield-Water' Relationships and Their Changes during Individual Climate Years. 17th Europ. Reg. Conf. on ICID, Varna, Bulgaria, May, pp. 351-360.