Gy. Kovács and A. Szöllösi-Nagy
Research centre for Water Resources Development
Budapest, Hungary
1. TYPE OF INFORMATION NEEDED FOR DESIGN AND OPERATION
2. HYDROLOGICAL FORECASTING FOR OPERATION PURPOSES
3. DETERMINING DESIGN PARAMETERS
4. DETERMINATION OF AREAL PRECIPITATION
5. THE REGIME OF SOIL MOISTURE
6. EVAPORATION AND EVAPOTRANSPIRATION
7. GROUNDWATER PROBLEMS ARISING IN CONNECTION WITH AQUACULTURE STRUCTURES
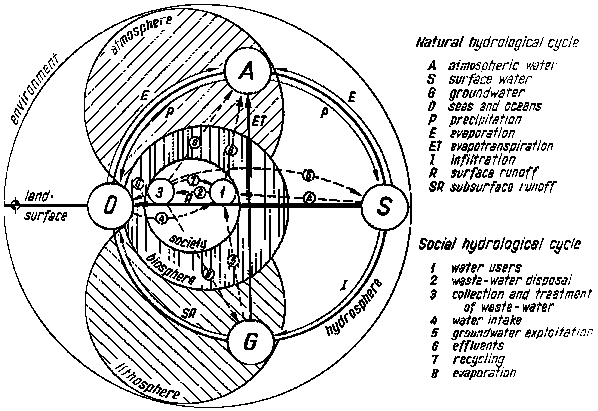
Water is one of the continuously renewable natural resources of the globe. The large cycle of the hydrosphere (the natural hydrological cycle) includes the evaporation of water from the surfaces of the oceans and the continents into the atmosphere, its return to the land-surface in the form of precipitation, and the surface and subsurface runoff conveying the water back to the lake basins, seas and oceans. It establishes a connection between the other spheres of the earth and it is an important component of the human environment (Figure 1). Economic development has created a second smaller cycle of water inside society. Water is taken out from natural resources, utilized in various forms and the effluents, having in most cases a modified quality, are released into the environment. The social cycle of water (water management) includes also those actions which modify the natural runoff to protect society against the harmful effects of water, including floods, erosion, and pollution.
Hydrology is a branch of water science describing the transport and storage of water through the cycles as well as the interactions between the water and its environment. Its task is to provide water management with information needed for designing and operating aquaculture structures and systems. These systems modify the natural water regime according to the requirements of society, to avoid damages and to best utilize the water. Hence all users of water, aquaculture among them, need hydrological information produced by collecting and processing hydrological data as well as by analysing the processes described by these data.
Considering the task of hydrology, it is evident that not only the natural processes have to be analysed, but the modification of the water regime due to human intervention must also be investigated. Data from both the natural hydrological cycle and the use of water in the social sphere, therefore, should be collected and evaluated. The description of a water regime includes not only the determination of the quantities transported and stored, but also the qualitative properties of water. Hence the hydrological information systems must provide data about the instantaneous condition of and the expected changes in water quantity and quality.
The information provided can be divided into two groups:
(i) Forecasts, prepared to inform the operators of existing hydraulic systems on the present condition of hydrological processes already in motion and the expected further development of these processes in a relatively short period (real time forecasting).(ii) Information required for planning, designing and constructing aquaculture structures and systems. This is composed of data on the extreme or average conditions of the hydrological processes expected to develop in the future without any limitation of time, other than the life span of the systems (design values).
In both cases forecasts have to be calculated from the hydrological data observed in the past. The basic difference between the two types of analysis is that in the first case the further development of a process started already is estimated and the actual time point of the occurrence of the predicted condition is determined, while the design values are calculated by using statistical methods and analysing long records without indicating the time of the development of the average or extreme conditions. Hence hydrological investigations providing the basis of forecasting, and the determination of design values, together with the utilization of their results, should be discussed separately.
There is another aspect which can be used to sub-divide the hydrological analysis, i.e., the character of the processes. From this point of view the analysis, and the application of the information is basically different depending on where and how the data are gathered:
(i) Transport processes developing in the network of rivers with data measured at sections of the river, which are used for problems of water conveyance, sediment transport, and transport of dissolved solids.(ii) Areally distributed processes maintaining water exchange between the various water horizons and measured in the form of point values within the catchment such as precipitation, evapotranspiration, and infiltration.
Figure 1. Natural and social hydrological cycles
Hydrological information needed for planning, design, construction and operation of any structures and systems of aquaculture is summarized in Table 1, which follows:
Table 1 Hydrological Information needed for Operation and Design of Aquaculture Systems
|
|
Areally distributed processes (vertical water exchange) |
Linear transport processes in rivers (horizontal transport) |
|
Information for the operation of aquaculture systems (real time forecast) |
quantitative forecast of precipitation |
continuous forecasting of discharge and water level |
|
|
forecast of critical meteorological conditions (e.g., storm forecasting) |
flood forecasting |
|
|
|
danger of accidental pollution |
|
Determination of design data for planning and design of structures and systems |
design parameters of climatic data |
mean and variance of both the multi-annual averages and the expected extreme values of discharge and water level |
|
|
area-depth-distribution of precipitation |
expected development of water quality and its relationship with water quantity and climatic data |
|
|
areal average of evapotranspiration |
|
|
|
regime of soil moisture |
|
|
|
balance of shallow ground-water |
|
2.1 Forecasting on Headwaters and Small Rivers
2.2 Forecasting the Regime of Large Rivers
Forecasts of flow volumes and water elevation are essential to making the most efficient use of rivers and in minimizing damage due to floods. On rivers uncontrolled by dams and reservoirs, the river forecast is the basis of flood warnings, permitting removal of people, livestock and movable goods from the flood plain, and reinforcement of fixed river structures, such as bridges. On rivers controlled or partially controlled by dams built for power production, water supply, irrigation or flood control, reliable river forecasts permit operation of the dams for maximum benefit. River forecasts are essential to the reconciliation of joint use of reservoir capacity for water supply and flood control purposes. The flood control capacity in some cases must always be available to store flood runoff, or in other cases the reservoir must be drawn down in advance of a flood, to a level which will permit accommodation of the anticipated flood volume. When a flood occurs the reservoir is restored to its normal supply level. Accurate forecasts are obviously essential because, if the reservoir is lowered too much and the water supply level cannot be restored after the flood, the water users may be short of water. Contrarily, if not lowered enough, more water may have to be discharged than would have been necessary and flood damage may result.
All potential users of rivers can benefit from reliable river forecasts. Efficient operation of multi-purpose reservoirs and issuance of flood warnings, navigation and pollution control benefits can accrue. On some rivers bearing heavy pollution loads, regulation agencies have insisted on many industries and municipalities developing storage facilities to enable them to discharge wastes into rivers only when flows are great enough to give safe dilutions of the effluent. Forecasts of likely flows a day or two in advance permit scheduling of effluent discharge to minimize storage requirements and still keep pollution concentrations below a safe level.
Hydrological forecasting is, by definition, the prediction of the occurrence of a hydrological event specified both with respect to its quantitive measure and its actual time of occurrence. The following classification is made according to the purpose of a forecast:
|
|
Quantity |
Quality |
|
Forecast of extreme situations |
Flood, low-flow, ice, droughts |
Sudden pollution |
|
Continuous real-time forecast of possible future states |
General purpose, for operation and control | |
On headwater tributaries and drainage areas smaller than a few thousand square kilometres, precipitation observations and predictions must be used to produce river forecasts early enough to be of value for reservoir operation, flood warnings, etc. On the other hand, for larger rivers with longer lag times, forecasts can often be based to a large extent on observed upstream flows.
For small drainage areas there are two main steps in the preparation of river forecasts. The first is to predict the volume of runoff by means of rainfall runoff correlations, and the second to forecast the distribution in time of the runoff volume. Rainfall runoff correlations can be derived from past records of storms and resulting river flows in a basin, either graphically or analytically. Such correlations permit forecasts of runoff volume from: (i) depth of storm rainfall over the watershed, (ii) a seasonal factor, (iii) an index of pre-storm moisture conditions in the basin, and sometimes (iv) storm duration.
Having determined the volume of rain and/or snowmelt which will run off, the timing of this runoff volume, and the peak flow that will result, must also be predicted. The unit hydrograph for the stream location in question is used to give the distribution of the runoff with time.
Different methods are used in preparing the unit hydrograph, such as:
- direct techniques (matrix method, use of orthogonal expansion, Fourier-series, etc.)- conceptual models (linear channels and reservoirs, Nash-cascade, soil-moisture accounting models, etc.)
For some purposes, such as reservoir operation, the unit hydrograph may be used to give a complete forecast of the rise and fall of a stream following a storm. For other purposes, such as flood warnings, and rapid forecasts to determine whether a given flood is likely to yield critical inflow rates to a reservoir, the important factors may be simply maximum stage and flow and the time at which they will occur.
It is possible to combine the rainfall-runoff correlations and the unit hydrograph peak values into a forecast graph which will give a rapid prediction of peak flow or stage. This is derived from the rainfall (or snowmelt) volume, the antecedent precipitation, a seasonal factor and the storm duration.
The trend in river forecasting is toward the use of physical parameters directly rather than through empirical correlations and index techniques. This has been made possible by the application of high-speed computers for rapid solution of more complex predictive equations.
Forecasts for large rivers and the lower reaches of principal tributaries generally make use of the dependable and consistent relationships between factors involved in stream-flow routing. The technique employed in a particular case depends upon whether the complete hydrograph is to be forecast or only the peak stage or discharge. On larger rivers the time between the end of the rainfall or snowmelt event and the occurrence of the peak of the resulting hydrograph at the point of interest is often measured in days, rather than hours as in headwater forecasting.
One of the simplest techniques in river forecasting makes use of the peak stage relation. This relation is easily constructed by plotting from past flood records the peak stages at the upstream station against the corresponding peak stages at the downstream station. The success of such a relation in providing an accurate estimate of the peak water level at a downstream station is dependent upon two assumptions: (i) the volume of inflow between the upstream and downstream station is small in comparison with the flow at the upstream station or is a constant proportion of that flow; and (ii) the peak of the local inflow always bears the same time relation to the peak of the flow at the upstream station.
A complementary relation is the time-of-travel curve which, as the name implies, provides an estimate of the time for the peak stage to travel from the upstream to the downstream station. The time of travel may vary directly with the stage throughout the range of stage or it may begin to increase more rapidly or less rapidly after a certain stage is reached depending upon the stream channel and valley characteristics.
Headwater hydrographs derived from rainfall-runoff correlations and unit hydrographs can be translated to downstream points on the main river by the techniques of streamflow routing. These techniques are essentially simplifications of the gradually varying unsteady flow equations.
An important application of hydrometeorology is the provision of criteria for the design of various water control structures, such as dams and storage reservoirs, storm sewers, bridges and irrigation systems. In order to determine the design values statistical techniques are used.
Hydrology and meteorology are concerned with natural phenomena, and the analysis of problems arising from these phenomena are dependent upon observations of their magnitude, duration and location. There will be little likelihood of obtaining successive measurements of the same event. Using statistical methods, a multiplicity of data can be reduced to manageable form and so enable the relevant data to be distinguished from the irrelevant. Statistical methods are particularly useful in checking the validity of a hypothesis involving complex natural phenomena. Having formulated an hypothesis, a statistical analysis of whether the observations fit that hypothesis would show whether or not there are good reasons to doubt it. Statistical analysis deals with probabilities and cannot provide conclusive proofs.
The procedures for estimating design values can be grouped as follows:
(i) Frequency analysis of flood flow records or frequency analysis of precipitation records and relating of precipitation to runoff from the catchment using the unit hydrograph or some other method.(ii) Transposition of storms.
(iii) Transposition of depth-area-duration relationships.
(iv) Probable maximum precipitation (PMP) method.
(v) Regional methods.
(vi) Empirical methods.
Determination of both probable maximum storm and standard project storm depends on the idea of storm transposition. The purpose of storm transposition is to increase the storm experience of a basin by considering not only storms which occurred in that basin, but also those which released their heaviest rainfall on adjacent areas that are meteorologically 'similar'. There are two difficult problems in storm transposition. The first is to define the region of meteorological similarity over which a particular storm can be transposed. The second concerns the permissible change in orientation of the storm rainfall pattern to yield critical runoff rates and volumes.
One of the design problems which is common to most uses of water is the degree to which the seasonal and annual fluctuations of streamflow can be modified by storage to correspond with the demand requirements; that is, the problem of gearing the supply to the demand by means of storage reservoirs. A reservoir design requires solutions to many problems.
The storage capacity of a reservoir may be required for one or several of the following uses: irrigation, municipal and industrial water supplies, power, flood control, sediment storage, navigation, recreation, and fish and wild-life habitat. However, in a given drainage basin the amount of storage available to serve one or a combination of these uses may be limited by economic considerations or by physical limitations such as topography, foundation strength for supporting a dam, and water supply. Economic factors involve such items as incremental costs of additional capacity, cost of right-of-way, cost of transportation facilities and many other factors peculiar to a particular reservoir location. Field surveys will provide data on reservoir capacity and reservoir area related to elevation of the water surface at the dam site. These non-hydrologic data are necessary for relating available supply to probable demands for the water.
The first hydrologic problem is to determine the supply of water that will be available for the proposed project. The best information on supply would be obtained from long-term flow records at the reservoir site. Records from a station a short distance upstream or downstream from the point in question would also be valuable. If adequate data are not available, the existing record can be extended or streamflow data synthesized.
An important consideration in determining reservoir capacity is the minimum annual runoff. The available storage determines the magnitude of demand that can be met during a period of low runoff. It is desirable, therefore, that the record or estimate of stream-flow volume embraces at least one dry period, which may encompass from one month to several years. Most reservoirs operate on an annual cycle, with each year's runoff, modified by storage, capable of meeting the demand. Some reservoirs are operated so that storage is carried over for several years to be certain of meeting a specific minimum demand even during a most severe drought.
In addition to deficient precipitation, drought may be associated with increased evapotranspiration losses. During such periods the flow in many drainage basins is from groundwater so that the flow in the stream is closely related to groundwater depletion. If the groundwater should drop below the bed of the stream, then the flow of the stream will cease except for surface runoff from snowmelt or intense local precipitation.
At high latitudes, minimum flows commonly occur during the months associated with long periods of freeze-up. The precipitation is held in storage in the form of ice and snow and the moisture in the soil layers may be frozen. In these regions temperatures are well below freezing over large areas, therefore runoff conditions also exhibit a fair degree of uniformity. This enables more than usually reliable comparisons to be made between adjacent streams and hence estimates of low flows at ungauged sites may be made more readily.
On lakes and reservoirs, both wind and differences in atmospheric pressure can cause changes in water levels which may seriously affect human safety, design and operation of dams, dikes, breakwaters, water intakes, and other lake structures, and the flow of outlet rivers. The wind exerts a horizontal stress on the water, raising the level from normal at the leeward shore and lowering the level on the windward shore. This is called a set-up effect which lasts as long as the force continues to be applied by the wind and is sometimes known as a wind tide. However, if the wind dies down quickly, or changes direction, an oscillatory motion takes place with alternate high and low levels being observed at both ends of the lake, with a periodicity characteristic of the dimensions of the water body. The oscillations are known as seiches.
The calculation of the magnitude of the initial set-up may be an important factor in assessing the amount of freeboard allowance required on dams, dikes, embankments or other retaining structures. It should be noted that set-up is greatest on long, shallow lakes and least on small, deep water bodies.
The transport by lake currents of water and materials in suspension and solution may have practical implications. Pollution from municipalities along lake shores and from ships on a lake will move with the currents. Under unfavourable conditions such pollution may enter water intakes. Currents also cause erosion of the shoreline. For these and other reasons, it is often necessary to know something of the currents in a lake or reservoir and the factors which cause them. The theory of currents in an infinite water body, applicable to the oceans, is also useful in dealing with lakes.
Wind-induced currents in a lake are closely interlinked with seiche and set-up effects because there must be currents to permit motion of large masses of water. When the wind which produces a set-up dies down, a current will flow from leeward to windward to restore the lake to its natural level. However, the current will continue due to its inertial energy and result in the temporary piling up of water at what was the windward end while set-up winds prevailed. This produces the seiche oscillations previously discussed. These oscillations are thus accompanied by currents, sometimes very strong ones.
Littoral drift is the transport, primarily by waves and coastal currents, of beach material along the shores of large lakes and reservoirs. As waves approach a coastline in a direction determined by the direction of the wind which generated them, they are refracted, since the portion of the wave first reaching shallow water slows down first. This tends to change the wave direction to one more parallel to the bottom contours and thus to the shoreline. However, this is rarely completely achieved and the breakers still retain a component of velocity along the shore, and shore currents are established. These currents can move eroded material along the shore.
Precipitation is the input to the continental branch of the hydrological cycle. Its best known forms are rain and snow, although there are areas where the amount of water reaching the surface in other forms (e.g. dew, fog, etc.) is not negligible either.
The amount of precipitation is measured by various standardized rain gauges, which might be supplemented with recording and telemetering devices. Without going into the detailed analysis of the reliability of gauge-readings, it is necessary to mention that there are systematic errors (due to evaporation, splashing, aerodynamic effects, wetting the instrument), apart from the random ones caused by erroneous readings or by the defects of instruments. The observed amount of precipitation is, as a rule, generally smaller than the true value.
In spite of the systematic errors of rain gauges the determination of the depth and intensity of precipitation at the observation points (point values) is more reliable than the measurements of other hydrological processes (e.g., river discharge or evaporation). More serious uncertainties are involved when the areal average of the precipitation is calculated, than those disturbing the basic data.
The information required for any hydrological study dealing with either surface run-off or groundwater regime is the total amount of precipitation reaching the ground surface of the catchment within the time interval investigated. The observation of precipitation provides, however, only point-values (the sample caught by one gauge having an orifice of 200 cm). The most important task of the hydrological analysis of precipitation data is, therefore, to calculate areal averages from gauge readings by constructing area-depth-distribution maps (Figure 2) and to consider the change of precipitation between the measuring level and the ground surface.
Considering the problems explained, the actual input of continental hydrological systems should be improved by ensuring a double transformation of the measured precipitation data. The most efficient increase of the accuracy of hydrological computation can be achieved by:
(i) Calculating the average of the areal precipitation from point values (investigating also the structural character of the field of precipitation); and(ii) reducing the measured values by considering the losses from precipitation between the measuring level and the terrain (characterization of interception).
The soil moisture zone is the heart of the continental branch of the hydrological cycle, because its structure and instantaneous condition determine the ratio of surface to subsurface runoff and even a considerable amount of evapotranspiration is also covered from the water stored below the terrain and above the water table.
The lower boundary of the soil moisture zone is the water table, which is a theoretical surface, where the total pressure prevailing in the water phase of the porous medium is equal to the atmospheric pressure. The excess pressure, which is the difference between total and atmospheric pressures, is, therefore, equal to zero. Hence the basic physical difference between soil moisture and groundwater is that the former is under suction because the pressure prevailing in the water above the water table is smaller than the atmospheric pressure.
It is necessary to consider that the water content of a porous medium is a function of the suction of the soil moisture. Hence both the storage capacity (soil moisture retention curve) and the transport capacity (unsaturated hydraulic conductivity) must be investigated depending on the suction distribution in the soil moisture zone. The field measurements should provide, therefore, information not only on the vertical distribution of moisture content, but the suction value must be measured at several points along the vertical profile. From this double observation both the change of the stored amount of water and the direction of the propagation of the moisture can be determined.
Figure 2. Area - depth - distribution map

In practice the information required most frequently for solving engineering problems includes the infiltration through the surface and the water exchange between soil moisture and groundwater (groundwater accretion). The former is needed to calculate surface runoff, which is the difference between precipitation reaching the terrain and infiltration. Ground-water accretion provides information on the vertical recharge and drainage of groundwater systems. Therefore both infiltration and groundwater accretion require the simulation of the complete soil moisture system (Figure 3) .
The detailed analysis of the regime of soil moisture has two main parts:
(i) The theoretical investigation should give methods to determine the interrelationships between suction and water content (soil moisture) retention curve or pF curve) as well as between suction and unsaturated hydraulic conductivity. Substituting these basic relationships into the transport equation (Richards equation) and combining the condition of continuity and the resistivity against the movement in unsaturated porous media in this way, the regime of the soil-moisture zone can be simulated. The model is suitable to determine any practical information required for hydrological studies such as infiltration, groundwater accretion, and instantaneous condition in and transport through the soil moisture zone.(ii) The practical part of the study deals with the observations and the measuring methods applied to determine the hydrological processes developing between the land-surface and the water table. The most important components are the vertical distribution of both the moisture content and tension, the amount of infiltration through the surface and the water exchange between soil moisture and groundwater.
Evapotranspiration is the main form of drainage from the continental branch of the hydrological cycle. Among its two components evaporation is basically a physical process of transforming a liquid into vapour. When this transformation is made by plants (transpiration) the process is influenced also by biological, biophysical and biochemical factors.
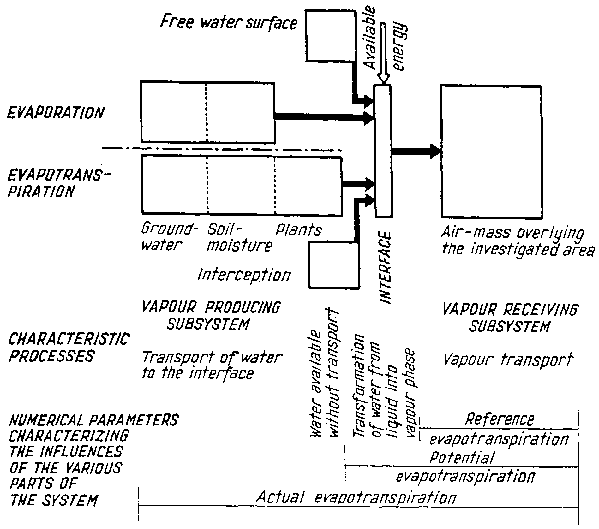
Unfortunately there is no direct method to measure the vapour flux, and indirect observations provide only point values similar to the measurement of precipitation. For the determination of the areal average of evapotranspiration the whole system transforming liquid water into vapour should be investigated (Figure 4). It is divided into two subsystems: (i) the vapour receiving air-mass; and (ii) the vapour producing terrain. The character of the interface between the two subsystems where the evapotranspiration actually occurs, and the amount of energy available here, are important factors in the system. The availability of water and the rate of saturation of the air may be also limiting factors.
To design and operate hydraulic structures and systems the areal average of actual evapotranspiration should be determined. For this calculation the amount of water actually drained from each part of the catchment having different surface-conditions should be estimated. Hence the investigation of evaporation and evapotranspiration includes the following:
(i) Measurement of evaporation from free water surface by use of evaporation pans. The determination of the pan-to-lake coefficient considering the oasis effect due to energy advection must be taken into consideration.(ii) The main components of evapotranspiration. To determine the best methods suitable for measuring evapotranspiration, distinction should be made according to the origin of water evaporated and transpired, i.e., interception, soil moisture, groundwater.
(iii) Methods to estimate actual evapotranspiration. The use of lysimeters, the measurement of the decrease of stored water and the calculation of evapotranspiration from the deficit of heat balance are investigated.
Figure 3. Soil moisture system

Figure 4. Evapotranspiration system
Groundwater, when it is under natural conditions, should be in balance, i.e., the multi-annual averages of its recharge and drainage respectively have to be equal. If not balanced, continuous rising or lowering of the water table would be observable. Any human intervention disturbing this equilibrium will have far reaching consequences. The exploitation of groundwater introduces a new drainage activity without changing the natural inputs and, therefore, it causes the continuing depletion of the groundwater resource. Aquaculture structures which raise the level of surface waters (e.g., dams or river barrages, canals, fish ponds) change the natural conditions, initiating artificial recharge or decreasing the natural drainage maintained by percolation from groundwater reservoirs to rivers. This change causes a rise in the water table until the development of a new equilibrium.
The design of aquaculture structures requires a forecast of expected changes in the groundwater regime due to the structures, followed by continuous observation after they are built. When the aquaculture structure modifies the movement of groundwater only in its close vicinity, the application of the principles of seepage hydraulics gives sufficient results, while more complicated groundwater systems have to be simulated to determine the far reaching effects of large engineering structures. In this case the hydrological processes interconnecting the groundwater systems with the surface and atmospheric branches of the hydrological cycle should be considered as boundary conditions.
For the investigation of complicated groundwater systems, it is necessary to include:
(i) The geological structure of aquifers and aquitards using geological mapping, geophysical prospecting and drilling.(ii) Hydraulic conductivity and storage coefficient, calculated from the physical parameters of the layers and measured either in laboratory or in the field.
(iii) The observation of potential-head by using test wells.
(iv) The boundary conditions influencing the development of seepage in the system.