The long-term objectives for fisheries management should take into consideration scientific fishing research and population dynamics, as well as the climatic changes that may affect the stocks.
In order to define these long-term objectives we have to consider the values of the fishing level, which allow bigger catches in weight, whilst also ensuring the conservation of the stocks. The extreme values of the biomass or the fishing level, which might seriously affect the self renovation of the stocks, also have to be considered. These fishing level values, of catch and biomass are designated as biological reference points (BRP). In this manual some of the different types of BRP will be considered (Caddy, & Mahon, 1995; FAO, 1996 and ICES, 1998).
The Target Reference Points, TRP are BRP defined as the level of fishing mortality or of the biomass, which permit a long-term sustainable exploitation of the stocks, with the best possible catch. For this reason, these points are also designated as Reference Points for Management. They can be characterized as the fishing level Ftarget (or by the Biomass, Btarget).
The most well known Ftarget is F0.1 but other values, like Fmax, Fmed, and FMSY will also be studied.
For practical purposes of management, the TRP will be converted, directly or indirectly, into values of fishing effort, given as percentages of those verified in recent years.
The Limit Reference Points, LRP are maximum values of fishing mortality or minimum values of the biomass, which must not be exceeded. Otherwise, it is considered that it might endanger the capacity of self-renewal of the stock.
In the cases where fishing is already too intensive, the LRP can be important to correct the situation or to prevent it from getting worse.
The LRP are limit values, mainly concerned with the conservation of marine stocks and they are therefore also referred to as reference points for conservation (this designation does not imply that the Ftarget are not concerned with conservation).
Several LRP have been suggested, which will generally be referred to as Flim or Blim. In this manual the levels of biomass Bloss and MBAL will be referred to as Blim and the fishing levels Floss and Fcrash as Flim.
The Precautionary Principle, proposed by FAO in the Conduct Code for Responsible Fisheries (FAO, 1995), declares that the limitations, uncertainties or lack of data for the assessment or for the estimation of parameters, cannot be justification for not applying regulation measures, especially when there is information that the stocks are over-exploited.
From this point of view, it is important to make clear which basic assumptions are necessary in order to estimate the consequences on the catches and on the abundance of the stocks.
The uncertainties associated with the estimation of Flim, and Blim, therefore lead us to determine new reference points, called Precautionary Reference Points, Fpa or Bpa.
The assumptions and the consequences of adopting alternative hypotheses about the stock and fishing characteristics should always be presented to justify the estimated values of Fpa (or Bpa).
The new limits (Fpa or Bpa) due to the application of the Precautionary Principle, will be more restrictive than the LRP's. The practical consequences of these new limits are the regulation measures designed to control the fishing effort which are more severe than in those cases where there is appropriate data.
It can be said that this is the price to pay for not having the appropriate conditions to make available reliable data and information.
The Precautionary Approach, suggests that the results of fisheries research should be adopted by management with regard to the formulation of the regulation measures and that these measures should also take into consideration the socio-economic and technical conditions of fishing (FAO, 1996).
A final remark about all the Biological Reference Points mentioned above:
The evaluation of the biological reference points has to be updated, taking into consideration the possible changes in the biological parameters or any other necessary correction of the exploitation pattern. This fact is important because the new biological reference points will be different from the previous ones.
5.2.1 Fmax
Definition
1. Consider the long-term yield per recruit, Y/R, as a function of F, for a certain exploitation pattern.
Fmax is the point of the curve, Y/R against F, where Y/R is maximum.
Figure 5.1 shows a curve of Y/R against F.
Figure 5.1 Y/R as function of F for a certain tc constant, showing Fmax and Ymax

In Chapter 4 it was mentioned that to estimate the long-term projections one could assume that the recruitment is constant and equal to 1 (R=1). In this way, the mathematical expressions are sometimes written with Y instead of Y/R.
2. Mathematically, at point Fmax, the derivative of Y/R against F is equal to zero, that is,
|
For F = Fmax will
be |
air(Y)=0 (Value of Y is maximum) |
|
For F < Fmax will
be |
air(Y)>0 (Y is increasing with F) |
|
For F > Fmax will be
|
air(Y)<0 (Y is decreasing with F) |
Geometrically, the slope of the tangent to the curve is equal to zero for F = Fmax, positive for F < Fmax and negative for F > Fmax.
Comments
1. 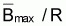 and
Ymax/R are the values at Fmax.
and
Ymax/R are the values at Fmax.
It is also convenient to analyse the situation of
 at the points F ≠
Fmax.
at the points F ≠
Fmax.
F < Fmax corresponds to
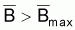
F > Fmax corresponds to
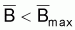
Point Fmax does not depend on the value of the recruitment.
2. For another relative pattern of exploitation there will be
another  .
.
3. All the points of the curve Y/R against F, are long-term points or equilibrium points.
4. When the level, F, is bigger than Fmax it is said that there is growth overfishing.
It is convenient to present the two curves Y/R and /R against F, in the same graph (usually with different scales).
Figure 5.2 Long-term curves of
Y/R and 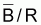 against F,
given an exploitation pattern
against F,
given an exploitation pattern
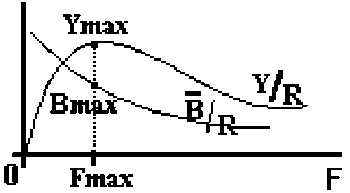
Fmax was adopted by the majority of the International Fisheries Commissions as a long-term objective of management (1950-1970).
Even today Fmax is used as a target-point having been proposed as a Limit Reference Point (LRP) in some cases.
The flat-top and asymptotical curves do not allow the determination of an Fmax.
The definition of Fmax does not consider the appropriate level of spawning biomass.
Fmax only indicates the value of F which gives the maximum possible yield per recruit from a cohort during its life, for a given exploitation pattern.
The analyses of these long-term curves, mainly of
 , Y and
, Y and
 against the fishing
level, give information about the abundance of the resource (or catch per
vessel), total yield of all the fleet and mean catch weight for different
fishing levels.
against the fishing
level, give information about the abundance of the resource (or catch per
vessel), total yield of all the fleet and mean catch weight for different
fishing levels.
5.2.2 F0.1
1. Definition
Consider the long-term yield per recruit, Y/R, as a function of the coefficient of fishing mortality, F. One value of air(Y/R), corresponds to each fishing level, F. The air(Y/R) is maximum when F = 0 and decreases, being zero when F = Fmax.
The point F0.1 is the value of F where air(Y/R) is equal to 10 percent of air(Y/R) maximum.
The figure 5.3 illustrates this situation.
Figure 5.3 Y/R showing the reference target point F0.1
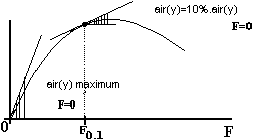
2. For F = 0, the biomass per recruit,
 will be
will be
 , also designated as
Virgin Biomass or Non-exploited Biomass. The air(y) at F=0 is also
equal to B0
, also designated as
Virgin Biomass or Non-exploited Biomass. The air(y) at F=0 is also
equal to B0
In fact, 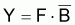 implies
implies 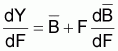
Then, for F = 0, air(Y) =

So, from the definition given in point 1 one can also say that F0.1 is the value of F where air(Y) = 10 percent of the virgin biomass.
3. Calculation of F0.1
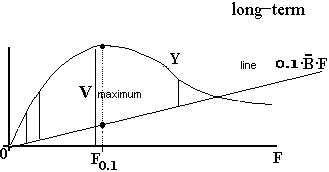
Let the function
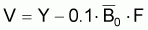
It can be proved that the function V is maximum when F = F0.1
In fact, V is maximum when
 , then:
, then:
or
Therefore, the value of F corresponding to the previous dY/dF is the value of F0.1.
F0.1 can then be calculated by maximizing the
function 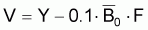
 can be
calculated, for example, from the long-term relation of
can be
calculated, for example, from the long-term relation of
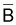 against F, when F =
0.
against F, when F =
0.
Graphically it will be:
Figure 5.4 Curve Y/R showing the maximum of the function V
4. Why adopt air(Y/R) equal to 10 percent and not any other percentual value, for example, 20 percent?
Gulland and Boerema (1969) presented some arguments, including financial arguments. Some countries (like South Africa) adopt the value of 20 percent with a resulting reference point F0.2 that is more restrictive than F0.1.
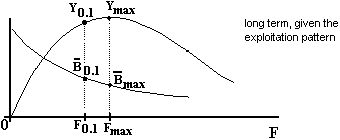
5. Figure 5.5 illustrates the two biological reference points Fmax and F0.1.
Figure 5.5 Long-term variation of Y/R and B/R against F and points corresponding to Fmax and F0.1
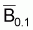 and are
the values of
and are
the values of 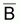 and
and
 corresponding to
F0.1
corresponding to
F0.1
F0.1 is always smaller than Fmax
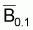 is always
larger than
is always
larger than 
Y0.1 is always smaller than Ymax, although, in practice, the difference is not large.
The second sentence above indicates the advantages of
 over Bmax. The
last sentence shows that Y0.1 is not the largest possible catch, but
is acceptable as a target point of management. The fact that
over Bmax. The
last sentence shows that Y0.1 is not the largest possible catch, but
is acceptable as a target point of management. The fact that
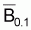 is larger than
max suggests that the fishing level F0.1 is preferable to
Fmax as TRP.
is larger than
max suggests that the fishing level F0.1 is preferable to
Fmax as TRP.
Notice that F0.1 can be calculated even when the curve is asymptotical or flat-top.
Another value of F0.1 will be obtained if the exploitation pattern changes.
In the years 1960-70 F0.1 started to be preferred to Fmax as a target point by resource managers, and it was adopted in the 80’s, as a long-term objective by many International Fisheries Commissions and by the EEC.
5.2.3 Fmed
1. Definition
This target point considers the relation S-R between the stock and the resulting recruitment, in order to avoid the assumption of a constant recruitment.
Let (the spawning or total) biomasses and the resulting recruitments for each year of a certain period of time be known. In this case, one can calculate the median value of the ratios between the annual spawning biomasses and the corresponding recruitments.
Fmed is defined as the F value corresponding to the median B/R ratio in the long-term B/R relation against F.
Usually, Fmed is illustrated by considering
the graph of the points corresponding to pairs of values of parental biomass
(total or spawning),
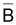 , during that year
and the resulting recruitment, R. Figure 5.6 shows this situation.
, during that year
and the resulting recruitment, R. Figure 5.6 shows this situation.
Figure 5.6 Illustration of a median line

The marked line is a line passing through the origin, which separates the total number of points in equal parts, that is, 50 percent of the points are in the upper part and 50 percent are in the lower part of the line. This line is designated as the median line, or 50 percent line, which can be explained as follows: in 50 percent of the years of the considered period the values of R were smaller than the values of R which were estimated by the median line (or, in 50 percent of the years of the referred period the values of R were bigger than the values of R estimated by the median line).
As seen in Section 4.5 the slope (B/R) of each line marked in the graph, is associated with a certain value of the fishing level, F. The value of F associated with the median line is then, the median target point, Fmed.
It can be said that, given a certain level of parental biomass
and knowing the 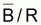 corresponding to Fmed, then there is a 50 percent probability that
the resulting recruitment will be less than (or greater than) the value
indicated by the median line.
corresponding to Fmed, then there is a 50 percent probability that
the resulting recruitment will be less than (or greater than) the value
indicated by the median line.
2. Calculation of Fmed
In order to determine the value of Fmed it is necessary to consider the long-term relation between the resulting biomass per recruit and the fishing level, F, (Section 4.4, Figure 4-D).
The determination of Fmed can be done mathematically or graphically.
To make the mathematical calculation of Fmed the
ratio 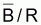 has to be determined
for each pair of values (B, R). Those values have to be ordered and then the
median value,
has to be determined
for each pair of values (B, R). Those values have to be ordered and then the
median value,  can be
calculated.
can be
calculated.
In the long-term relation of the Biomass per Recruit against F, the value of Fmed is the value of F that corresponds to the median value previously obtained.
To make the graphical calculation, notice that in Figure 5.6
the slope of the median line against axis R is equal to
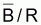 . This value will be the
basis for the calculation of value Fmed in the graph of
. This value will be the
basis for the calculation of value Fmed in the graph of
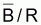 against F, in the long-term
projection. Figure 5.7(A-B) illustrates the calculation.
against F, in the long-term
projection. Figure 5.7(A-B) illustrates the calculation.
Figure 5.7 Illustration of the graphical calculation of Fmed
|
A)
|
B)
|
Other straight lines, corresponding to probabilities other than 50 percent, can be marked. Figure 5.8 illustrates the graphical calculation of F10%. The line marked on Figure 5.8A, separates the points, in such a way that 10 percent of them remain below the line (or 90 percent of the points stay above the line). So, this line has been designated as the 10 percent line.
Notice that the slope of the 10 percent line with axis R is larger than the slope of the median line, as shown in Figure 5.9B. In this way, F10%, or Flow, is smaller than Fmed.
Figure 5.8 Illustration of the graphical calculation of F10%
|
A)
|
B)
|
Figure 5.9 Graphical illustration of the slopes and corresponding F's for the median and 10 percent lines
|
A)
|
B)
|
Comments
1. The target point Fmed intends to ensure an acceptable level of biomass based on the empirical relation S-R.
2. Other percentages can also be adopted, corresponding to straight lines which estimate different probabilities of recruitments which are less than those indicated by the median line. So, Fhigh would be the fishing level corresponding to the 90 percent line, for which the recruitments of 90 percent of the observed years would be less than those estimated by the line.
3. F90%, as will be seen in the following Section, is also considered as a Limit Point (LRP).
4. Notice that the slope of the 10 percent line with axis R is larger than the slope of the median line and therefore, F10% (or Flow) is smaller than Fmed (Figure 5.9B).
5. Fmed was used in management, in recent years, particularly with Iberic sardines.
6. The biomass used can be the total biomass,
 , but is frequently the
spawning biomass, SP.
, but is frequently the
spawning biomass, SP.
7. If the median line does not pass through a marked point in the scatter plot (which happens when there is an even number of points) then one can use any straight line passing between the two central points, for instance, the mid-line. In any case, Fmed is always an approximate value.
5.2.4 FMSY
Definition
FMSY is defined as being the value of F which produces the maximum yield in the long-term. It is necessary to select an S-R relation to estimate FMSY. This point is different from Fmax.
There are several proposals for Flim and Blim. For each stock, the adopted values of Flim and Blim depend on the characteristics of the stock and on its exploitation. What is important is that the adopted LRP be a value that allows an exploitation level which avoids dangerous situations of stock renewal.
Some of these points are derived from the observed values of Biomass and of Resulting Recruitment. Some examples of this type are Bloss and MBAL. These LRP are also usually classified by some authors as non-parametric, because their determination does not depend on any particular model of the S-R relation.
Another category of LRP points, classified as parametric, is derived from S-R models.
Fcrash will be mentioned.
Let us also mention the category of LRP points involving observed values and values obtained by the application of S-R models, like, for example, Floss.
5.3.1 Bloss
Bloss is the smallest spawning biomass observed in the series of annual values of the spawning biomass (Lowest Observed Spawning Stock).
5.3.2 MBAL
More satisfactory is the LRP designated as Minimum Biological Acceptable Level, MBAL. In fact, this LRP is a spawning biomass level below which, observed spawning biomasses over a period of years, are considered unsatisfactory and the associated recruitments are smaller than the mean or median recruitment.
5.3.3 Fcrash
The name itself shows that it is a limit that corresponds to a very high value of F, showing a great probability of collapse of the fishery.
Fcrash is the fishing level F which will produce a long-term spawning biomass per recruit (S/R) equal to the inverse of the instantaneous rate of variation of R with the biomass, at the initial point (S = 0, R = 0). With the S-R models of Section 4.5 that value is the parameter 1/a of the models.
In order to make the graphical determination of this LRP one
can start by obtaining the slope of the angle that the tangent to the S-R curve
makes with the R axis at the origin. Afterwards, and starting from the relation
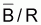 against F, the value of F
that corresponds to the value
against F, the value of F
that corresponds to the value
 indicated by that slope is
obtained. Fcrash will then be the value of F corresponding to
indicated by that slope is
obtained. Fcrash will then be the value of F corresponding to
 equal to that slope, in the
long-term relation
equal to that slope, in the
long-term relation  against
F.
against
F.
5.3.4 FLOSS
Floss is usually defined as the fishing level F which will produce a long-term spawning biomass per recruit (S/R) associated to Bloss.
To determine this limit point, first obtain the value of R corresponding to Bloss on the adjusted curve S-R. Then, calculate Bloss/R and find the value of F, in the long-term relation B/R against F.
Most of the Limit Points shown have been criticised for depending on the observed values or on the adjustment of the S-R relation.
As previously mentioned, the Precautionary Principle recommends that the assessments should be done even when the basic data presents some gaps. This recommendation implies that, in this case, the determination of the Biological Reference Points will not be very precise. The uncertainities of the estimates should be calculated, and it is necessary to mention the assumptions and models which have been used.
One suggestion to determine Fpa and Bpa might be to estimate Flim or Blim and from these values, to apply the following empirical rules:
Fpa = Flim·e-1.645.σ and Bpa = Blim·e+1.645.σ
The constant σ is one measure associated with the uncertainity in the estimation of the fishing mortality level, F. The values obtained in several fisheries indicate that values of s are within the interval (0.2, 0.3) (ICES, 1997). In practice, it can be said that Fpa is between 0.47Flim and 0.61Flim, and Bpa is between 1.39Blim and 1.64Blim.
It is important to make clear that the target points may also, in certain cases, be considered as limit or precautionary points depending on the combined analyses of the exploitation of the stock and of the biological reference points obtained.
The regulation measures aim to control the fishing level and the exploitation pattern applied to the stock for an adequate exploitation.
The most common regulation measures to control fishing levels are:
TAC is a measure that directly controls the catch and, indirectly, the fishing level. It is convenient to combine the TAC with the allocation of quotas of this total TAC for each component of the fleet. In this way, the competition between vessels to fish the maximum possible catch, as quickly as possible, before the TAC is reached, can be avoided.
The system of quotas allocated to each vessel is called Individual Quotas (IQ).
The regulation measures to correct the exploitation pattern are usually called technical measures. Some of these measures are:
The fishing management have the duty to promote legislation and the application of the regulation measures. (In the particular case of the EU, and for the stocks of the Economic Exclusive Zones (EEZ's) of the member states, the Commission decides on the measures to be taken). In any case, management needs the analyses on the state of the stock and its exploitation and on the effects of the recommended measures. That study must be done by the fisheries scientists of each country or region and their Fishing Research Institutes, who will have to calculate the projections of the stocks. The International Council for the Exploitation of the Sea (ICES) analyses the assessments and recommends regulation measures and the expected effects of the application of those measures to the Commission, as well as the consequences of their non-application.
The short-term projections, as well as the regulation measures, only make sense if the long-term objectives of fisheries management are previously analysed and defined. Short-term projections of the stock and of its fishing must also be made by the scientists.
Comments
1. Management needs to define the fishing objectives, based on the long-term projections. Those objectives are valid for a period of years, even if they can be adjusted every year.
2. The regulation measures, on the contrary, have to be established every year, although some of them may be valid for more than one year. Some technical measures, like the minimum mesh sizes of the fishing nets or the minimum size of the landed individuals, are valid for several years.
3. All the measures have advantages, difficulties and disadvantages regarding the purposes they intend to reach.
The concession of fishing licences is a common practice almost everywhere, with a limited total number.
TACs and quotas, because they control the catches, have caused misleading declarations about catches.
The direct limitation of the total fishing effort (f), is based on the assumption that the measure causes a similar limitation on the fishing mortality coefficient (F). However, this relation may not be proportional. In the first place, it is difficult to measure the fishing effort of the different fishing gears and of all the involved fleets and it is also difficult to express it in units that respect the proportionality between F and f. Secondly, the capturability of several gears may increase (and consequently increase F) without increasing the fishing effort. Finally, the expected proportionality between F and f may not be true. In any case, what matters, is not to forget that there is a relation between F and f.
4. The protection of the juveniles should be carried out during the whole year and will preferably control the fishing mortality throughout the year. The occasional measures, like areas and periods when fishing is forbidden for the protection of juveniles, require annual investigations in order to discover whether there are exclusive concentrations of juveniles, to assess the effects of that occasional interdiction, and to find out the consequences of the interdiction on other species, etc. The minimum size of the landed individuals, does not mean that smaller individuals are not caught, but only that they are not landed. The difference between the catch and the landing is the so-called rejections to the sea. It is clear that if the individuals are caught and rejected to the sea, the fishing mortality is larger than the one suggested by the landings. The minimum landing size of the individuals may have the effect of dissuading fishermen from catching small individuals. Currently, some countries are forcing the landing of all fish caught.
5. The closed spawning areas and seasons, to save the spawning biomass and indirectly protect recruitment, is far from effective in the latter objective. In fact, large spawning biomasses correspond to a large number of eggs, but that does not necessarily imply bigger recruitments, as seen in Section 4.5. It is also not always true that forbidding fishing during the spawning, and not forbidding before (or after) the spawning, protects the spawning biomass. The only way to protect the spawning biomass will be to control fishing level during the whole year. Finally, it has to be said that, in any case, the interdiction of fishing in the spawning area and period, or on any other occasion, always represents a reduction of the fishing effort. This is not a major inconvenience and in some cases, may even be beneficial.
6. It has to be stressed that no regulation measure will accomplish its objectives without observing two conditions:
The understanding of the fishermen (broadly speaking) that the measure is good for the fishery. Hence, it is important to discuss the scientists' conclusions, their objectives, their reasons and the expected effects.
An efficient fiscalization in the ports and at sea! The 200-mile exclusive zone may be very vast and the fiscalization very expensive, but it is not necessary to fiscalize the whole area intensively. It is enough to control the areas of larger catches more intensively and the remaining areas less so.
During the last few years, new ways of controlling access to fisheries resources and exploitation levels are being implemented. Some examples are the establishment of Individual Transferable Quotas systems (ITQ), co-management systems or even the system of regional or municipal management, where some management responsibilities are attributed to the resource users themselves.
7. The ITQ management system is based on the abusive assumption that only the economically efficient and profitable vessels deserve to be active in fishing. So, TAC's are divided into individual quotas, to be auctioned for the best offer.
The co-management system delegates a great part of the responsibility of management to those who directly exploit the fishing resources - managers, fishermen and their professional organizations or unions. With this system neither are quotas sold in auctions, nor are the fishing licences lost.
These are the most well known systems.
The ITQ system presents the following inconveniences: permanent loss of the titles of quotas and of fishing licences; concentration of quotas in the hands of a small group of people (who may not even belong to the fishing sector or are even foreigners); and underestimation of the social, human and cultural aspects, in favour of economic efficiency criteria.
On the contrary, the co-management system, is concerned with the social aspects of the people involved; it seeks their direct and conscientious co-participation with government authorities in the management responsibilities, including fiscalization.