6.1 Data for mortality rates and population size
6.2 Varying distribution in space
6.3 Exercises
Tagging of fish can be used in studying growth, and movements and migration. This section, however, is concerned only with the use of tagging data to determine the mortality rates of the population, and the size of the population. (Growth is referred to in section 3.) The simplest assumption is that the tagged fish are subject to constant fishing and natural mortality rates which are the same as in the natural untagged population, and these can be estimated separately, because, unlike the natural population, the initial number in the tagged population is known precisely.
That is, if N0 fish are tagged at a certain moment, the number Nt alive after time t is given by
..................... (6.1)
The rate at which these are caught is equal to the fishing mortality coefficient times the number alive and is therefore given by
where n = number of tagged fish recaptured.
The total number of returns from t = 0, the time of tagging up to time T is therefore obtained by integrating, i.e.
If the returns are grouped in time intervals of length T, the number nr caught during the rth interval between times rT, (r + 1) T is therefore
...........(6.2)
(Note: The first interval after tagging is r = 0.)
The number caught in successive intervals can therefore be used, knowing N0, to give estimates of F and M. In particular,
... (6.3)
so that if log nr, is plotted against r, the result is a straight line, slope - (F + M) T. (An alternative and rather more convenient form of this equation is given in equation [6.9].) Using this value of (F + M), F can be estimated from the numbers returned in any interval, using equation (6.2), or from the intercept of the y-axis, which from equation (6.3) is
Alternatively, the total number of returns up to time t is
from which, substituting for F + M, a direct estimate of F can be obtained. When t is very large this reduces to 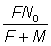 .
.
Unfortunately the assumptions made on this simple example are not often fulfilled. The types of errors which may arise can be placed in definite groups, according to their effect on the various estimates.
(a) Errors which affect the estimate of the rate of fishing (F), but not the estimate of total mortality (Ricker's type A, Beverton and Holt's type 1):(i) death of fish immediately after tagging;(ii) incomplete reporting of tags recaptured by fishermen (provided the proportion not reported stays constant; a variable reporting rate would also affect the estimate of total mortality).
(b) Errors which affect the estimate of the total mortality, but not that of fishing (Ricker's type B, Beverton and Holt's type 2):
(i) loss of tags from fish which occurs at a steady instantaneous rate throughout the experiment;(ii) additional mortality of tagged fish, also occurring throughout the experiment;
(iii) emigration of tagged fish.
© Errors affecting estimates of both fishing and total mortality:
(i) tagged and untagged fish not equally vulnerable to fishing, e.g. the additional likelihood of fish tagged with Petersen disks to be retained by a net;(ii) tagged fish not uniformly mixed with the untagged population.
Type (a) errors will lead to an underestimate of the true fishing mortality, which in itself may often be useful. They can often be detected and possibly measured by suitable observations. Thus in suitable conditions tagged fish can be kept in tanks or cages and their mortality for the period after tagging can be directly observed. Less directly the percentage returns of fish which when tagged were in different conditions - lively or not so lively, damaged or apparently undamaged, etc., or captured by different methods - can be compared. If there is any mortality due to the shock of capture and tagging then it will presumably be lower for lively fish, and higher for bruised or damaged fish (loss of scales often appears to be particularly critical). Absence of such difference can be taken as an indication, though not proof, of little or no such mortality.
Nonreporting of tags can often be detected by comparing the number of tags returned per unit quantity of fish caught by various groups of vessels fishing in the tagging area - individual boats, different countries, etc. It may be directly measured by feeding known numbers of tagged fish into the catches; this is general practice with mechanical methods of recovery (e.g. magnets at fish meal factories), but can, particularly with the co-operation of a few fishermen, be used for normal external tags returned by fishermen or shore workers.
Type (b) losses can be considered as an additional cause of mortality, i.e. loss of individuals from the population of tagged fish, so that equation (6.1) should be written as
.................... (6.4)
where X includes all causes of reduction of tagged fish other than fishing - loss of tags and emigration as well as natural mortality. Equation (6.4) and the corresponding form of equation (6.2)
......... (6.5)
will be the usual form for analysis of tagging data, so that in fact only the fishing mortality of the natural population will be estimated from tagging. Natural mortality will be given by subtraction from the total mortality, Z, estimated from age composition, etc.
Some quantitative estimate of type (b) losses can sometimes be obtained, for instance losses of tags (but not mortality of tagged fish) can be estimated by attaching two tags to each fish and noting the proportion of fish returned with both tags, and with only one tag, and the change in this proportion with time.
Differential vulnerability to fishing of tagged fish can usually be eliminated by suitable design of tags. Fish carrying button-type tags are likely to be particularly vulnerable to certain types of gear - e.g. set nets - and if possible such tags should not be employed where this type of gear is used, but if they have to be used, or in order to interpret past data, the extent of the extra fishing mortality can be gauged by comparing the rate of return of fish with different types of tags.
A distribution of tagged fish different from that of untagged fish is likely to present one of the major problems. If mixing is relatively quick, returns during some initial period, 0 to t', during which the tagged fish are mixing with the untagged population, may be omitted in the computations. The analysis is then carried on from time t', with a reduced initial number of tags, using equation (6.1) in the form
.................. (6.6)
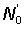 is estimated from the number tagged, subtracting the number returned in the initial period of mixing, and the estimated number lost through other causes, X. This last estimate will have to come from an analysis of the later data. However, in many situations nearly all returns will be made before the tagged and untagged populations are even approximately mixed.
is estimated from the number tagged, subtracting the number returned in the initial period of mixing, and the estimated number lost through other causes, X. This last estimate will have to come from an analysis of the later data. However, in many situations nearly all returns will be made before the tagged and untagged populations are even approximately mixed.
This mixing may be effectively speeded up by a suitable pattern of tagging. The aim is to have the ratio of tagged to untagged fish the same throughout the population. This might for instance be achieved by making an evenly spaced grid of trawl stations covering the whole area, and tagging all, or a constant proportion of, the fish caught at each station. This is possible in a small area but not easy to do in, say, the North sea.
In a large area such as the North sea it may not be possible to ensure effective mixing of tagged and untagged fish, or at least by the time the mixing has occurred most of the returns have taken place, and to ignore returns occurring before mixing was effectively complete would mean discarding most of the available data. However, if good information is available on the geographical distribution of the fishing effort, and on the positions of the capture of the returned tags, useful estimates of the fishing mortality on the untagged population can be made. The logical steps are:
(a) Estimate the fishing mortality, F, on the tagged population.(b) From the distribution of fishing effort and of the tagged fish estimate the fishing intensity, f, on the tagged population.
(c) Hence estimate q, in the relation F = qf.
(d) Estimate the fishing intensity on the whole population.
(e) Hence, assuming that q for tagged and untagged fish is the same, estimate the fishing mortality on the whole population.
It should be noted that, especially in the first steps, it is not necessary to consider fishing by all vessels, or in all areas, provided the same section of the total is used in computing fishing mortality (from returns of tags) and fishing intensity (from data on fishing effort). For instance, in the North sea some of the most extensive detailed data on fishing effort is of fishing by United Kingdom deep-sea trawlers; it therefore may be convenient to compute the fishing mortality and intensity only from the data of United Kingdom trawlers, and ignore returns of tags, and fishing activity by United Kingdom seiners and inshore vessels, and by vessels of other countries.
Consider a small area around the position of tagging in which mixing of tagged and untagged fish takes place quickly. For a certain time interval, i, let
= mean number of tagged fish present
fi = fishing intensity (effort per unit area per unit time)
ni = number of tagged fish returned.
Then
...................... (6.7)
If then 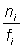 , the tags returned per unit fishing intensity, is plotted fi against time, and a curve fitted to the points, the intercept of this curve on the y-axis will be qN0, where N0 is the number released. Hence q can be estimated and the fishing mortality on the population as a whole will be given by
, the tags returned per unit fishing intensity, is plotted fi against time, and a curve fitted to the points, the intercept of this curve on the y-axis will be qN0, where N0 is the number released. Hence q can be estimated and the fishing mortality on the population as a whole will be given by
where 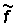 is the effective overall fishing intensity on the population, as calculated from detailed effort statistics.
is the effective overall fishing intensity on the population, as calculated from detailed effort statistics.
While the following assumptions may not be completely justified, to a first approximation the fishing intensity within the small area chosen around the release point may be taken as constant, and the movement out of this area defined by a constant exponential coefficient, D, and therefore the number of tagged fish in the area after time t, Nt, will be given by
where F is the fishing mortality occurring in the area around the release point, and not necessarily that in the population as a whole. If the ith interval is of duration T, and extends from time 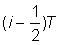 to
to 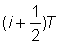
or
...............(6.8)
Thus, so long as (F + X + D)T is small, i.e. the numbers do not change very greatly during one interval, to a close approximation the term in the brackets will be equal to unity
................ (6.8')
and the plot of 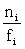 against time should be a straight line if plotted on a logarithmic scale. It may be noted that equation (6.8), and particularly its approximate form (6.8') suggests a good alternative to equation (6.3) in the form
against time should be a straight line if plotted on a logarithmic scale. It may be noted that equation (6.8), and particularly its approximate form (6.8') suggests a good alternative to equation (6.3) in the form
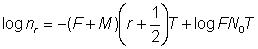
(6.9)
where again the last term may be neglected if the change in numbers during the interval is small, i.e. if the logarithm of numbers recaptured during each interval is plotted against the midpoint of the interval, then the plot should be a straight line, slope - (F + M) T, and intercept on the y-axis log FN0T.
Equation (6.7) can be extended to cover data from more than one small area around the release point. Denoting values for particular areas by prefixes
when 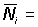 total of tagged fish in the areas being analysed
total of tagged fish in the areas being analysed
and therefore
............... (6.10)
so that the analysis can be carried out as before, using the sum of the tags returned per unit fishing intensity in each small area. The analysis can be carried out only when sufficient effort data are available; if tags are returned from a mixed fleet, some of which provide adequate effort data and some of which do not, then in all the analysis only data from the fleet or fleets providing complete data both of effort and tags returned should be used. Tagged fish caught by the other fleet are considered as other losses. Data from the other fleet are brought into the considerations only at the end, when computing the fishing mortality on the population as a whole.
1. Graham (1938) tagged 1 000 cod in the North sea, and the following numbers were returned, grouped in three-monthly intervals.
|
Months |
0-2 |
3-5 |
6-8 |
9-11 |
|
Numbers |
139 |
91 |
52 |
40 |
By plotting the logarithm of the numbers returned against time, estimate the total and fishing mortality coefficients. (Compare the results of using equation [6.3] and the approximate form of equation [6.9].)
2. Whiting tagged and released in the Irish sea were graded according to their liveliness and the damage to their scales, and the numbers released and subsequently recaptured were as follows (data from Beverton and Bedford, 1963).
|
Condition |
Scales |
Number tagged |
Number returned |
|
Lively |
Good |
60 |
18 |
|
Lively |
Moderate |
98 |
21 |
|
Lively |
Poor |
49 |
6 |
|
Sluggish |
Good |
254 |
60 |
|
Sluggish |
Moderate |
765 |
159 |
|
Sluggish |
Poor |
509 |
43 |
What evidence is there of mortality at tagging? Assuming all the best quality fish survived, what proportion of each other category died, and what was the effective number released?
3. From cod tagged in the Gulf of Saint Lawrence in 1955, the following numbers were returned by different countries in 1956 (from Dickie, 1963).
|
Country |
Number of tags |
Total cod landings |
|
|
|
tons |
|
Canada |
392 |
62400 |
|
Prance |
32 |
28000 |
|
Portugal |
29 |
5 800 |
|
Spain |
15 |
8 100 |
Calculate the number of tags returned per 1 000 tons of fish landed by each country. What evidence is there of incomplete returns? If all tags recaptured by Canadian fishermen are returned, estimate the number actually recaptured by other countries.
4. Three groups of herring tagged with internal tags were released in the North sea in the summer of 1957. Detailed data of Danish fishing intensity in the areas around the release points were available, in terms of hours' fishing per statistical square (15 × 15 miles).
The number of tags returned, and the fishing intensity around each tagging position in the weeks following tagging, were as follows (data adapted from International Council for the Exploration of the Sea, 1961).
|
Week after tagging |
Liberation number |
|||||
|
1 |
2 |
3 |
||||
|
|
Tags |
Intensity |
Tags |
Intensity |
Tags |
Intensity |
|
1 |
21 |
80 |
80 |
420 |
3 |
10 |
|
2 |
35 |
64 |
12 |
60 |
3 |
5 |
|
3 |
30 |
53 |
10 |
50 |
8 |
15 |
|
4 |
8 |
26 |
2 |
10 |
2 |
10 |
|
5 |
10 |
56 |
1 |
10 |
- |
- |
|
Number tagged |
3 000 |
1 500 |
3 000 |
|||
Plot the tags returned per hours fishing per square for each liberation. Hence, if the efficiency of the recovery technique is such that 90 percent of the tags caught are returned, what is the value of q, in terms of an intensity of 100 hour's fishing per square per week?